15 Jan – Harald Helfgott: Expander graphs in number theory: the prime divisibility graph
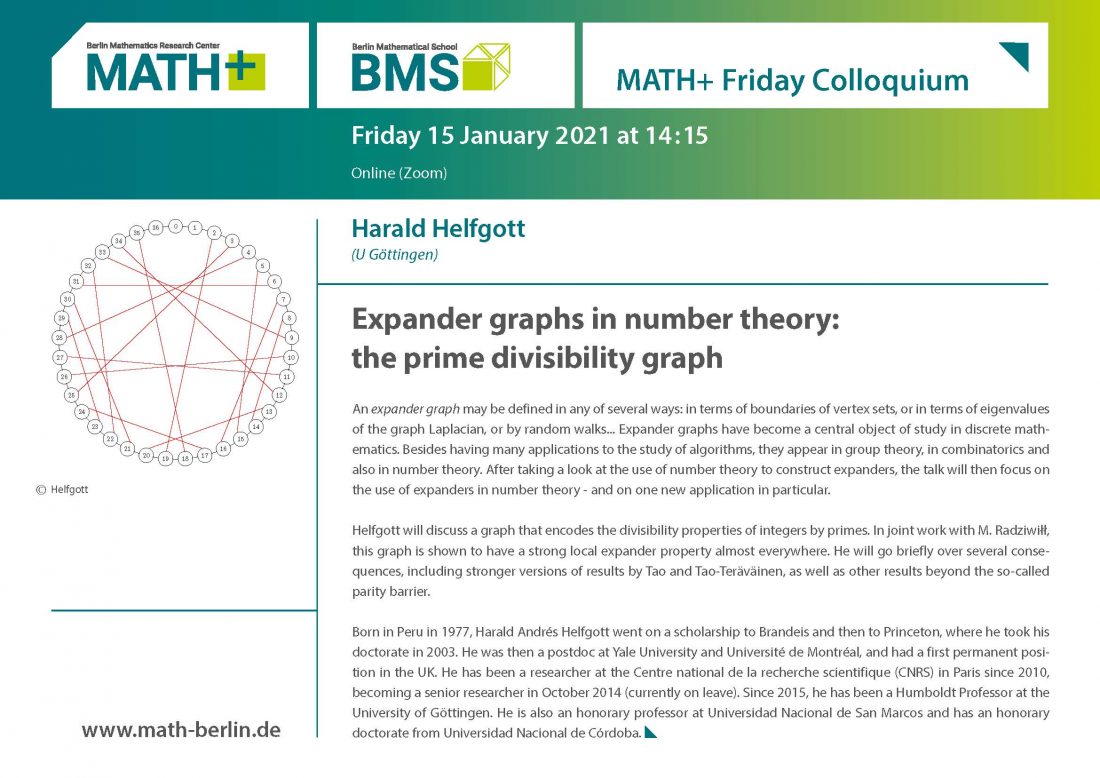
An expander graph may be defined in any of several ways: in terms of boundaries of vertex sets, or in terms of eigenvalues of the graph Laplacian, or by random walks… Expander graphs have become a central object of study in discrete mathematics. Besides having many applications to the study of algorithms, they appear in group theory, in combinatorics and also in number theory. After taking a look at the use of number theory to construct expanders, the talk will then focus on the use of expanders in number theory – and on one new application in particular.
Helfgott will discuss a graph that encodes the divisibility properties of integers by primes. In joint work with M. Radziwiłł, this graph is shown to have a strong local expander property almost everywhere. He will go briefly over several consequences, including stronger versions of results by Tao and Tao-Teräväinen, as well as other results beyond the so-called parity barrier.
Born in Peru in 1977, Harald Andrés Helfgott went on a scholarship to Brandeis and then to Princeton, where he took his doctorate in 2003. He was then a postdoc at Yale University and Université de Montréal, and had a first permanent position in the UK. He has been a researcher at the Centre national de la recherche scientifique (CNRS) in Paris since 2010, becoming a senior researcher in October 2014 (currently on leave). Since 2015, he has been a Humboldt Professor at the University of Göttingen. He is also an honorary professor at Universidad Nacional de San Marcos and has an honorary doctorate from Universidad Nacional de Córdoba.